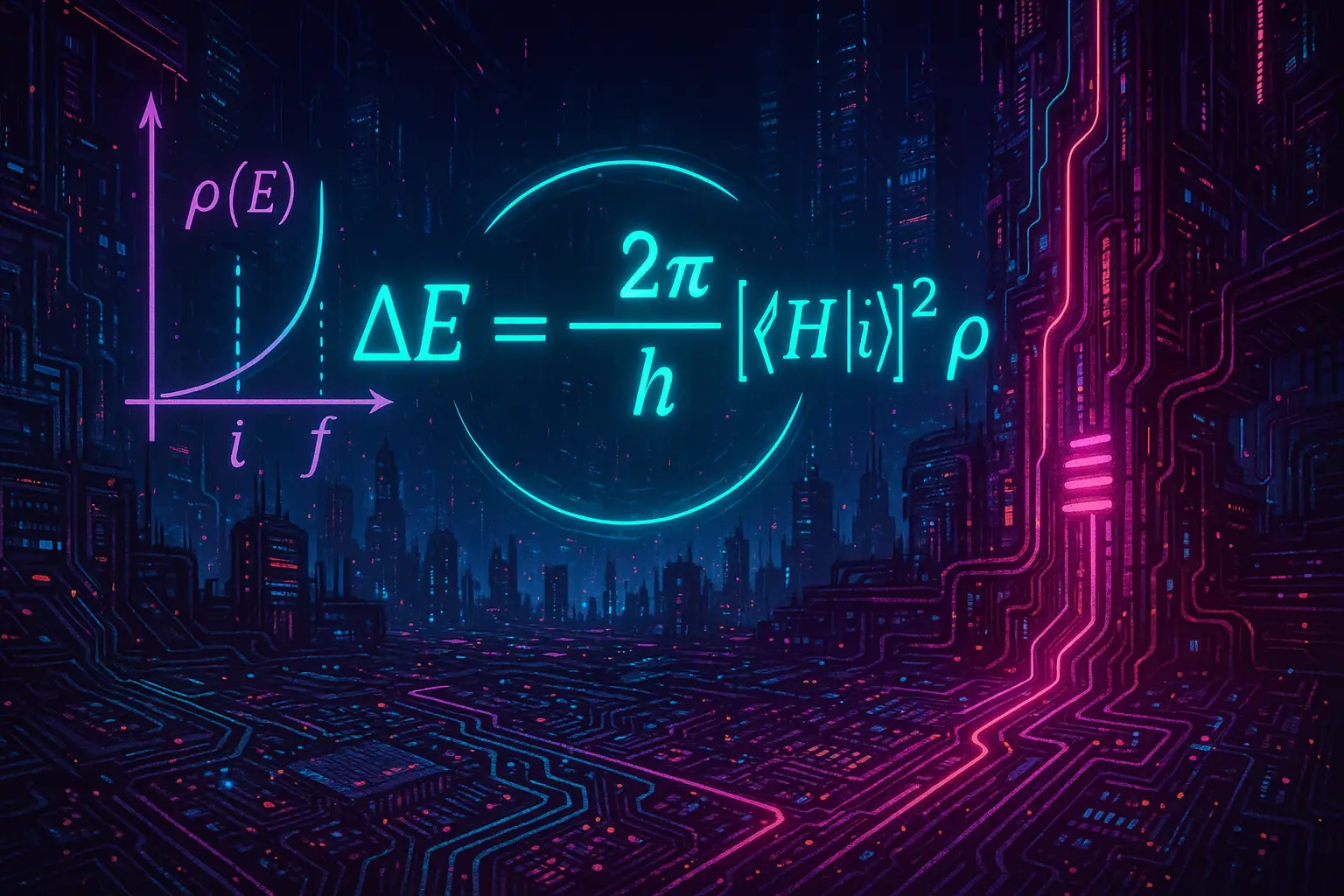Table of Contents
- Introduction
- Historical Background and Significance
- Motivation for the Rule
- Derivation from Time-Dependent Perturbation Theory
- Mathematical Expression
- Transition Rate and Density of States
- Physical Interpretation
- Validity Conditions
- Example: Spontaneous Emission of a Photon
- Example: Particle Scattering in a Potential
- Comparison with Classical Rates
- Applications in Atomic, Nuclear, and Condensed Matter Physics
- Limitations and Cautions
- Variants and Extensions
- Conclusion
1. Introduction
Fermi’s Golden Rule provides a formula for computing the transition rate between quantum states under the influence of a weak time-dependent perturbation. It is one of the most widely used results in quantum physics, particularly in atomic transitions, scattering theory, and decay processes.
2. Historical Background and Significance
Enrico Fermi developed this rule in the 1930s while analyzing beta decay. It helped bridge quantum theory with measurable decay rates and laid the foundation for the quantum theory of radioactive processes. Today, it is indispensable in both theoretical derivations and experimental calculations.
3. Motivation for the Rule
When a system is exposed to a weak perturbation, it may transition from an initial quantum state \( |i\rangle \) to a final state \( |f\rangle \). The goal is to compute the transition probability per unit time (i.e., rate) for this process, particularly when the final states form a continuum.
4. Derivation from Time-Dependent Perturbation Theory
Assume a perturbing Hamiltonian of the form:
\[
\hat{H}(t) = \hat{H}_0 + \lambda \hat{H}'(t)
\]
Let \( \hat{H}'(t) = \hat{V} e^{-i\omega t} + \hat{V}^\dagger e^{i\omega t} \), and suppose \( \hat{H}'(t) \) couples the initial and final states. From first-order time-dependent perturbation theory, the transition amplitude is:
\[
c_f(t) = \frac{1}{i\hbar} \int_0^t \langle f | \hat{H}'(t’) | i \rangle e^{i\omega_{fi} t’} dt’
\]
The probability is then:
\[
P_{i \to f}(t) = |c_f(t)|^2
\]
5. Mathematical Expression
Fermi’s Golden Rule gives the transition rate \( \Gamma \) as:
\[
\Gamma_{i \to f} = \frac{2\pi}{\hbar} |\langle f | \hat{H}’ | i \rangle|^2 \rho(E_f)
\]
Where:
- \( \Gamma_{i \to f} \): Transition probability per unit time.
- \( \langle f | \hat{H}’ | i \rangle \): Matrix element of the perturbation.
- \( \rho(E_f) \): Density of final states at energy \( E_f \).
6. Transition Rate and Density of States
The density of states \( \rho(E) \) measures how many quantum states exist per unit energy near a given energy level. In systems where the final states form a continuum, this density is essential to compute the transition rate:
\[
\rho(E) = \frac{dn}{dE}
\]
7. Physical Interpretation
- The transition rate is proportional to the square of the coupling strength between initial and final states.
- It is also proportional to the number of available final states.
- Intuitively, stronger interactions and more available final states result in higher transition probabilities.
8. Validity Conditions
Fermi’s Golden Rule applies under the following conditions:
- The perturbation is weak.
- The perturbation is time-independent or slowly varying.
- The final state is part of a quasi-continuum.
- The interaction time is long enough for energy conservation to emerge via the delta function \( \delta(E_f – E_i – \hbar\omega) \).
9. Example: Spontaneous Emission of a Photon
An excited atom decays to a lower energy level and emits a photon. Fermi’s Golden Rule yields the spontaneous emission rate:
\[
\Gamma = \frac{2\pi}{\hbar} |\langle f | \hat{d} \cdot \vec{E} | i \rangle|^2 \rho(\omega)
\]
Where \( \hat{d} \) is the dipole operator and \( \vec{E} \) is the electric field mode.
10. Example: Particle Scattering in a Potential
A particle incident on a scattering center may be deflected. The potential acts as a perturbation \( \hat{H}’ = V(\vec{r}) \). Fermi’s Golden Rule computes the differential cross-section by integrating over all final momenta.
11. Comparison with Classical Rates
Unlike classical physics, which may assume deterministic transitions, quantum rates depend on probability amplitudes and the interference of different quantum paths. Fermi’s Golden Rule quantifies these inherently probabilistic effects.
12. Applications in Atomic, Nuclear, and Condensed Matter Physics
- Atomic transitions (optical and microwave frequencies).
- Nuclear decay rates.
- Semiconductor band transitions.
- Phonon emission and absorption in solids.
- Quantum tunneling rates and ionization processes.
13. Limitations and Cautions
- Not valid for short times or strong perturbations.
- Assumes non-degenerate initial states unless modified.
- Energy conservation is enforced statistically over time, not instantaneously.
- Corrections needed for resonant or divergent behaviors.
14. Variants and Extensions
- Golden Rule for degenerate states.
- Time-dependent generalizations for pulsed interactions.
- Extensions in many-body and field-theoretic contexts (e.g., Fermi’s Golden Rule in QED).
15. Conclusion
Fermi’s Golden Rule is a cornerstone of quantum transition rate calculations. By linking microscopic interaction strengths with macroscopic transition probabilities, it serves as a bridge between quantum dynamics and observable decay and scattering phenomena. Mastery of this concept is essential for physicists across all specializations of quantum theory.