Table of Contents
- Introduction
- What Are Pauli Matrices?
- Matrix Definitions
- Algebraic Properties
- Commutation and Anticommutation Relations
- Eigenvalues and Eigenvectors
- Geometrical Interpretation
- Pauli Matrices and Spin Operators
- Rotations in Spin Space
- Pauli Matrices in Quantum Gates
- Pauli Matrices and the Bloch Sphere
- Pauli Matrices in Tensor Products
- The Pauli Group
- Pauli Matrices and Quantum Measurements
- Applications in Quantum Mechanics and Computing
- Conclusion
1. Introduction
The Pauli matrices are a set of three \( 2 \times 2 \) complex Hermitian and unitary matrices that play a foundational role in quantum mechanics. They are used to describe spin-½ systems, form the algebra of SU(2), and are key components in quantum computing for defining qubit operations.
2. What Are Pauli Matrices?
Pauli matrices arise naturally when describing the spin of quantum particles like electrons. They are used to represent the spin operators for spin-½ particles and define the fundamental algebra of the SU(2) Lie group, which underpins angular momentum in quantum mechanics.
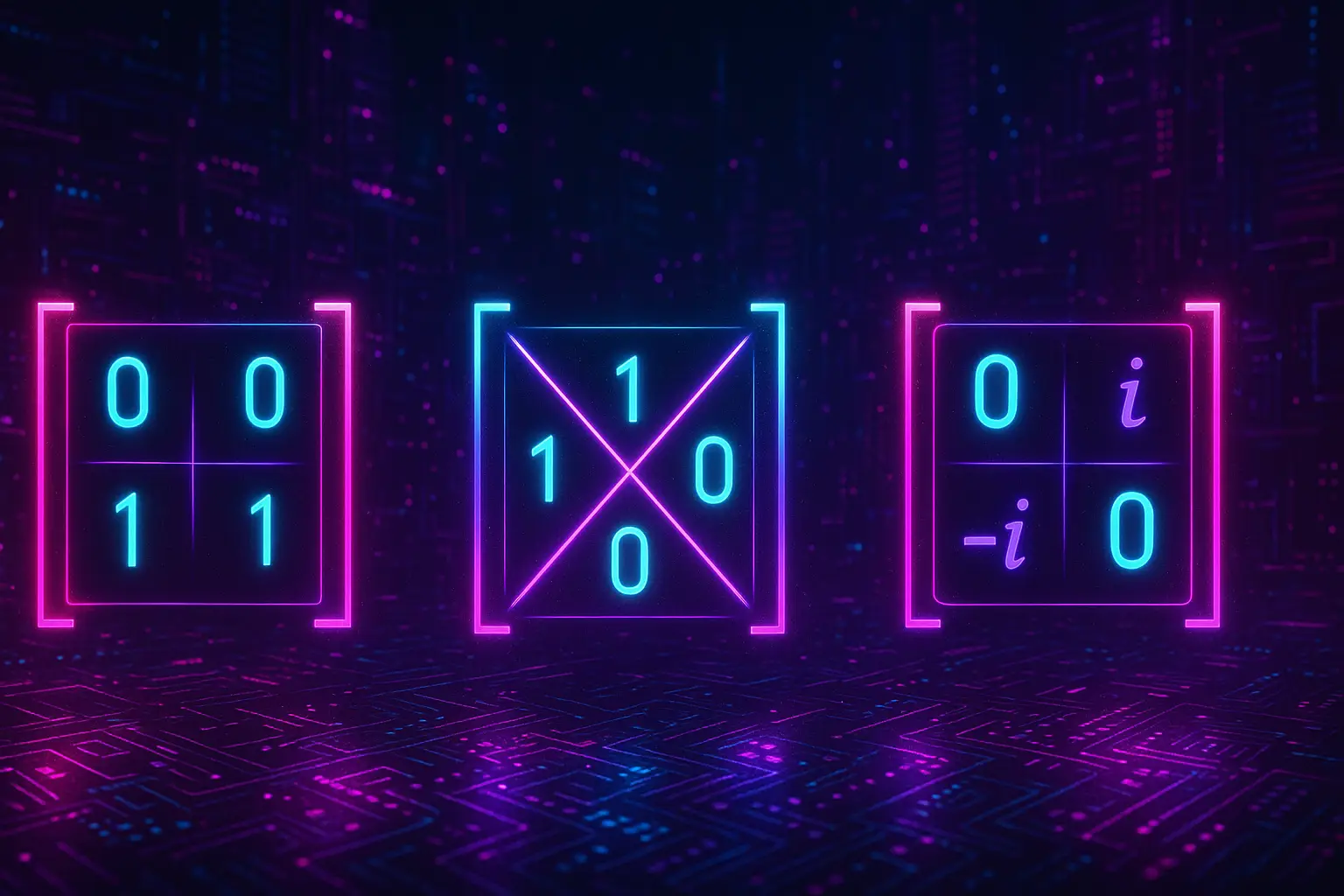
3. Matrix Definitions
The three Pauli matrices are:
\[
\sigma_x = \begin{pmatrix} 0 & 1 \ 1 & 0 \end{pmatrix}, \quad
\sigma_y = \begin{pmatrix} 0 & -i \ i & 0 \end{pmatrix}, \quad
\sigma_z = \begin{pmatrix} 1 & 0 \ 0 & -1 \end{pmatrix}
\]
They are often accompanied by the identity matrix:
\[
I = \begin{pmatrix} 1 & 0 \ 0 & 1 \end{pmatrix}
\]
4. Algebraic Properties
- Hermitian: \( \sigma_i^\dagger = \sigma_i \)
- Unitary: \( \sigma_i^\dagger \sigma_i = I \)
- Traceless: \( \text{Tr}(\sigma_i) = 0 \)
- Determinant: \( \det(\sigma_i) = -1 \)
5. Commutation and Anticommutation Relations
The Pauli matrices obey the following relations:
Commutation:
\[
[\sigma_i, \sigma_j] = 2i \epsilon_{ijk} \sigma_k
\]
Anticommutation:
\[
{\sigma_i, \sigma_j} = 2\delta_{ij} I
\]
Where \( \epsilon_{ijk} \) is the Levi-Civita symbol and \( \delta_{ij} \) is the Kronecker delta.
6. Eigenvalues and Eigenvectors
Each Pauli matrix has eigenvalues \( \pm 1 \). For example:
- \( \sigma_z \) eigenstates:
\[
\sigma_z |0\rangle = +1 \cdot |0\rangle, \quad
\sigma_z |1\rangle = -1 \cdot |1\rangle
\]
These states correspond to spin “up” and “down” along the z-axis.
7. Geometrical Interpretation
Pauli matrices can be viewed as generators of rotations in a 2D complex vector space:
- \( \sigma_x \): rotation about the x-axis
- \( \sigma_y \): rotation about the y-axis
- \( \sigma_z \): rotation about the z-axis
These rotations are visualized using the Bloch sphere.
8. Pauli Matrices and Spin Operators
Spin-½ angular momentum operators are defined as:
\[
\hat{S}_i = \frac{\hbar}{2} \sigma_i
\]
This allows the use of Pauli matrices to model quantum spin and calculate expectation values, dynamics, and observables.
9. Rotations in Spin Space
A rotation of a spinor by angle \( \theta \) about axis \( \hat{n} = (n_x, n_y, n_z) \) is given by:
\[
U(\theta, \hat{n}) = \exp\left( -i \frac{\theta}{2} \vec{n} \cdot \vec{\sigma} \right)
\]
This is central in describing how qubit states evolve on the Bloch sphere.
10. Pauli Matrices in Quantum Gates
In quantum computing, Pauli matrices define the Pauli gates:
- \( X = \sigma_x \): NOT gate (bit flip)
- \( Y = \sigma_y \): Bit and phase flip
- \( Z = \sigma_z \): Phase flip
These form the basis of many quantum algorithms and circuits.
11. Pauli Matrices and the Bloch Sphere
Every single-qubit pure state can be represented as:
\[
|\psi\rangle = \cos\left(\frac{\theta}{2}\right)|0\rangle + e^{i\phi} \sin\left(\frac{\theta}{2}\right)|1\rangle
\]
This state maps to a point on the Bloch sphere with angles \( (\theta, \phi) \), and Pauli matrices determine its evolution and measurement projections.
12. Pauli Matrices in Tensor Products
For multi-qubit systems, tensor products like:
\[
\sigma_x \otimes I, \quad \sigma_y \otimes \sigma_y
\]
Describe operations on composite systems. These are essential in entanglement, quantum teleportation, and quantum gates like CNOT.
13. The Pauli Group
The Pauli group is formed by all products of Pauli matrices with phases \( \pm1, \pm i \):
\[
\mathcal{P}_n = { \pm I, \pm iI, \pm \sigma_x, \pm i\sigma_x, \dots }
\]
Used in quantum error correction and stabilizer codes.
14. Pauli Matrices and Quantum Measurements
Measurements along an axis \( \hat{n} \) correspond to projecting onto eigenstates of:
\[
\hat{n} \cdot \vec{\sigma} = n_x \sigma_x + n_y \sigma_y + n_z \sigma_z
\]
This enables measurement of spin and polarization in any direction.
15. Applications in Quantum Mechanics and Computing
- Spin dynamics and magnetic resonance
- Qubit operations in quantum algorithms
- Quantum state tomography
- Quantum cryptography (BB84 protocol)
- Bloch equations in quantum optics
16. Conclusion
Pauli matrices are simple yet powerful tools in quantum theory. They form the algebraic backbone of spin-½ systems and single-qubit operations. Their elegant structure enables analysis of quantum states, dynamics, measurements, and transformations in both foundational physics and cutting-edge quantum technologies.