Table of Contents
- Introduction
- What Is Phase Space?
- Coordinates in Phase Space
- Trajectories and Hamiltonian Flow
- Volume Elements and Liouville’s Theorem
- What Is Symplectic Geometry?
- The Symplectic Form
- Canonical Transformations and Symplectic Structure
- Poisson Brackets and Symplectic Geometry
- Darboux’s Theorem
- Symplectic Manifolds in Higher Dimensions
- Applications in Physics
- Conclusion
1. Introduction
Classical mechanics is often introduced in terms of position and velocity. But to fully capture the structure of dynamical systems — and transition smoothly to quantum mechanics — we need a more refined view. That’s where phase space and symplectic geometry come in.
These concepts allow us to treat motion geometrically. Instead of tracking a particle along a path in space, we follow its state in phase space, where all possible positions and momenta are mapped out.
Symplectic geometry provides the mathematical structure underlying this space — a geometry of areas, not distances.
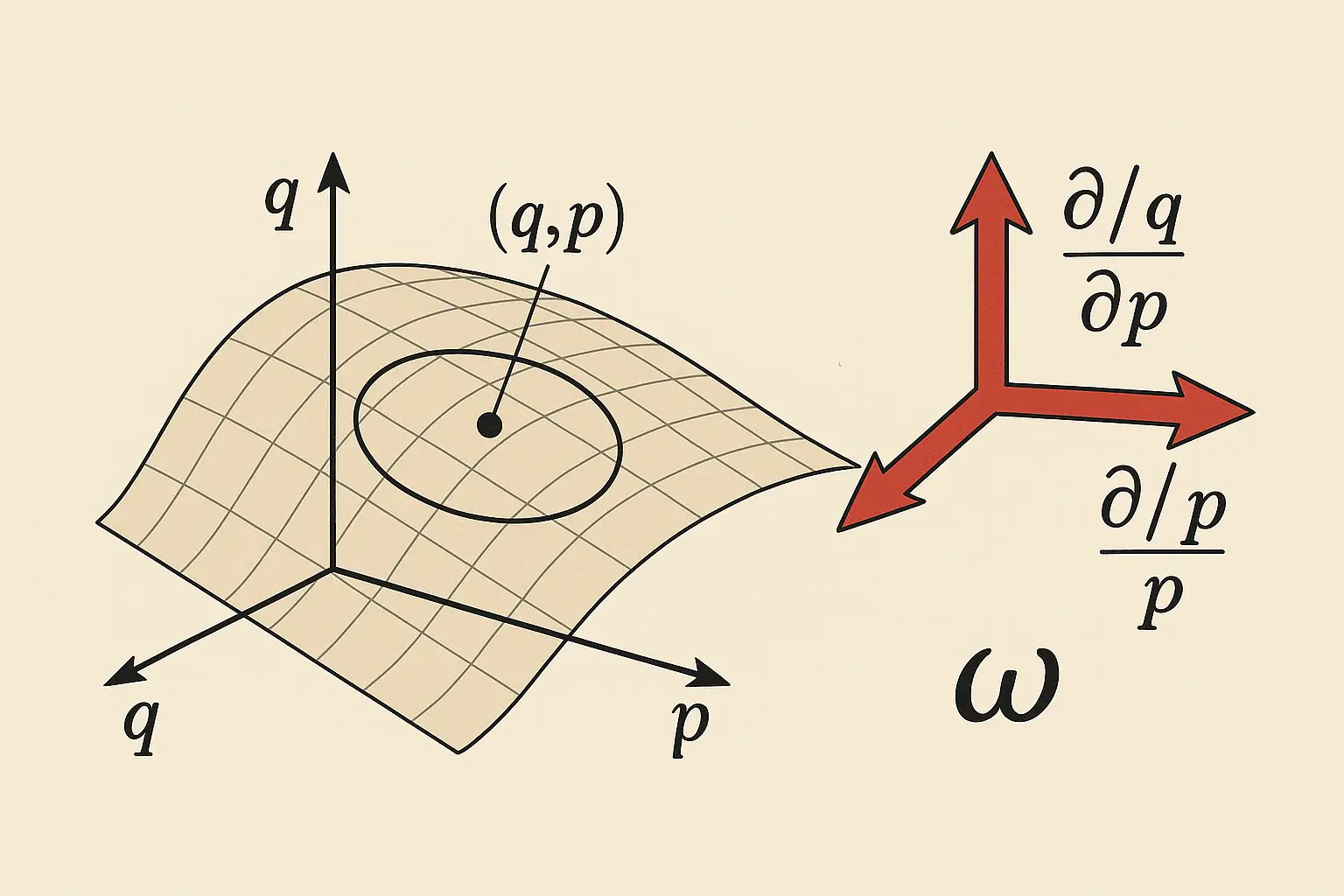
2. What Is Phase Space?
Phase space is a space where each point represents a complete state of a physical system.
For a system with \( n \) degrees of freedom:
- Position coordinates: \( q_1, q_2, …, q_n \)
- Momentum coordinates: \( p_1, p_2, …, p_n \)
The full state is a point in the \( 2n \)-dimensional phase space:
\[
\text{Phase space vector: } \mathbf{z} = (q_1, …, q_n, p_1, …, p_n)
\]
Each trajectory in phase space corresponds to the evolution of a system over time.
3. Coordinates in Phase Space
For a simple one-dimensional system:
- Position: \( q \)
- Momentum: \( p \)
The phase space is the \( (q, p) \)-plane. The evolution of the system is a curve in this plane.
In general, for \( n \) degrees of freedom, we need \( 2n \) coordinates.
4. Trajectories and Hamiltonian Flow
The motion in phase space is governed by Hamilton’s equations:
\[
\dot{q}_i = \frac{\partial H}{\partial p_i}, \quad \dot{p}_i = -\frac{\partial H}{\partial q_i}
\]
These define a vector field on phase space — the direction and speed at which the system evolves. A trajectory is an integral curve of this field.
5. Volume Elements and Liouville’s Theorem
In phase space, we can define a volume element:
\[
dV = dq_1 \wedge dp_1 \wedge \dots \wedge dq_n \wedge dp_n
\]
Liouville’s theorem states:
The volume in phase space is preserved under Hamiltonian flow.
This expresses conservation of information — the “density” of phase points does not change over time.
6. What Is Symplectic Geometry?
Symplectic geometry is a branch of differential geometry that focuses on the structure of phase space. It deals with non-degenerate, closed 2-forms, especially the symplectic form, which encodes the structure of classical mechanics.
Symplectic geometry is the natural setting for Hamiltonian dynamics.
7. The Symplectic Form
The canonical symplectic form in \( 2n \)-dimensional phase space is:
\[
\omega = \sum_{i=1}^n dq_i \wedge dp_i
\]
This form captures the geometry of the phase space. It is:
- Non-degenerate: Ensures invertibility between positions and momenta.
- Closed: \( d\omega = 0 \), which underpins conservation laws.
8. Canonical Transformations and Symplectic Structure
A canonical transformation is one that preserves the symplectic form:
\[
\omega’ = \omega
\]
This means the new coordinates still satisfy Hamilton’s equations and maintain the same physical content. Such transformations are also called symplectomorphisms.
9. Poisson Brackets and Symplectic Geometry
Poisson brackets arise naturally in symplectic geometry. For two functions \( f \) and \( g \), their Poisson bracket is:
\[
\{f, g\} = \sum_i \left( \frac{\partial f}{\partial q_i} \frac{\partial g}{\partial p_i} – \frac{\partial f}{\partial p_i} \frac{\partial g}{\partial q_i} \right)
\]
This is equivalent to:
\[
\{f, g\} = \omega(X_f, X_g)
\]
where \( X_f \) is the Hamiltonian vector field associated with \( f \). This shows that Poisson brackets are rooted in the geometry of symplectic space.
10. Darboux’s Theorem
A powerful result in symplectic geometry is Darboux’s Theorem:
Any symplectic manifold is locally equivalent to standard phase space.
This means that locally, every symplectic manifold can be described with coordinates \( (q_i, p_i) \) where the symplectic form takes its standard form.
There is no “curvature” in symplectic geometry — all local symplectic spaces look alike.
11. Symplectic Manifolds in Higher Dimensions
In multi-particle systems, phase space becomes very high-dimensional.
- 3D particle: 6D phase space
- Two particles in 3D: 12D phase space
- Fields: Infinite-dimensional symplectic manifolds
Despite the complexity, the symplectic structure provides the organizing principle for dynamics.
12. Applications in Physics
Symplectic geometry appears in:
- Classical mechanics: Structure-preserving transformations
- Quantum mechanics: Geometric quantization, phase space formulations
- Statistical mechanics: Liouville measure for ensembles
- Celestial mechanics: Integrable systems, Poincaré sections
- Field theory: Hamiltonian formulations of electrodynamics, general relativity
- Geometric optics and control theory
13. Conclusion
Phase space and symplectic geometry provide a powerful lens through which to view classical mechanics. They replace vector-based equations with geometric flows, emphasizing structure and conservation.
Their concepts are fundamental not only in classical systems but also in the foundations of modern physics, including quantum theory, relativity, and beyond.